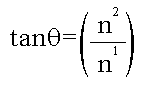Brewster's Angle
When Sir David Brewster was studying Polarization he found that at a certain angle the reflected light was completely polarized. We now call this light s-polarized. The p-polarized light is completely transmitted throught the medium.
As holographers we use this method to polarize lasers by inserting a brewster's window into a laser or to minimize the reflections from a piece of glass holding our emulsion.
Since out laser is completely polarized we can choose s or p polarization independently from the other.
A graph of reflection vs incedent angle for s-polarized (dashed Line) light and p-polarized (solid line) light.
Brewster's Law
Brewster's Law calculates the angle of minimum reflection for p-polarized light. n1 and n2 are the refractive indicies of the two media. Usually for us that is 1 for air and about 1.5 for glass.
So, for air at 1.00029 and glass at 1.5 we get:
tan(theta)=1.5/1.00029
theta=56.3deg
And then for gelatin 1.36:
tan(theta)=1.36/1.00029
theta=53.66deg
More information about when to use Brewster's Angle can be found here.
Finding Brewster's Angle
One way to determine Brewster's Angle is to set up your laser for p-polarization and place a single piece of glass in it. Hit the glass with your spread beam. The glass is going to reflect some of the light hitting it, so place a white card in this reflected light path (in order to view it). If you rotate your glass plate, you will notice that this reflected light becomes brighter and dimmer. Find the spot within the rotation where the reflected light is at it's dimmest on your white card, and you've got it. With a properly-running diode, the reflection will go completely out on the card (100% -- or VERY close to 100% -- transmission through the glass).
Also of interest is the angle change when a beam travels between two materials of different indicies of refraction defined by Snell's Law.